Understanding rivers

For an obscure reason I’m trying to understand rivers and particularly the changes in width, depth & velocity along a river.

Introduction

For anyone new to rivers, the important property of rivers is that water having once joined a river tends to keep flowing down the river. Therefore

Q = w d v
(where Q is flow, w average width, d average depth, v average velocity

Of course the very terms “average … ” width, depth, etc are complicated for rivers. However, the usual meaning is that if we take a cross section of area A (which is equal to average depth x average width)  and we measure the velocity and flow through this cross section then:

Q = A. v

Thus for a river at constant speed,

w = Q/d

Thus going down the same stretch of river, we tend to see wider-shallower sections and deeper, narrower sections.

Similarly for a river of constant width

v ∝ Q / d

Meaning that shallow sections tend to be faster (aka rapids)

And for constant depth

v ∝ Q / w

Thus when we have a “spout” where the channel is squeezed, we tend to see an increase in speed.

However, these changes require another variable to change. In this case, the prime variable that allows these changes, is a change in the river slope.

The following article explores the above relationships when the river slope is not seen as an independent variable changing to facilitate the above, but instead I attempt to understand the situation when the slope is controlling the above. This makes the whole concept far more complex, as there is no longer any “freedom” within the system. For slope to change, something else must in turn give – so we can no longer make simple assumptions.

River Relationship

In general terms the hydrology of a stream is governed by simple relationship:

Energy gained from going downhill = Energy going to increased  water velocity + Energy lost to heat (through turbulence).

And the energy lost to turbulence per unit length in a channel can be equated with:

frictional roughness of the channel bottom and sides x channel “wetted perimeter”.

Thus, in general, because channels on higher slopes gain more energy per unit distance travelled, this must be offset by having a rougher channel, consisting of larger stones or bedrock, OR by tending to have a relatively wider channel with a larger contact area with the turbulent (energy losing) bottom and sides

However, this obvious “bed roughness” of larger particles is not the only way that kinetic energy gets turned into heat. Such losses of forward energy can occur whenever the laminar flow of a river is caused to be turbulent. One common example of this is when the river turns a corner. In order to turn the corner, there must be an inward force, effectively pushing from the bank toward the centre of the turn. But because water is not a solid, this force is exerted as a pressure gradient. And this in turn is expressed as change in river surface level. Thus, the outside of a bend will be marginally higher than the inside. We can easily see this effect if we stir a cup of water – the water “creeps” up at the sides and depresses in the middle in the well known shape of a whirlpool.

However, whilst this pressure gradient is sufficient to turn the “average” part of the river, it has different effects on water that is flowing faster or slower than average. Water near the surface tends to move faster than water near the bottom of a river. So surface water is faster than average and bottom flows slower. But because the inward force needed is proportional to velocity squared,  and because the whole column experiences the same pressure, the pressure gradient is TOO LOW to turn the faster flowing water near the top of the river and TOO HIGH for the slower water near the base.

As a result, the pressure is insufficient to turn the faster moving surface water so that it tends to move outward from the centre of bulk flow on corners, whilst the lower water moves inward toward the centre. This creates a “corkskew” like vortix – whilst helps move bottom particles in toward the centre, deepening the outside of the bend, and making the inside shallow.

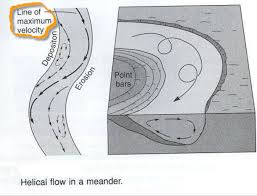However, when the river stops turning … because the water has momentum, it continues in its helical flow. Something which cannot stop until this helical flow gives rise to subsidiary vortices, and those in turn to others until the whole flow becomes a mass of whirling vortices, which quickly diminish in size until they are no different from the internal molecular movement known as heat.

Thus, merely turning a corner, can create a vortex which creates a mechanism to turn the energy gained in forward movement into heat. In other words, turning corners slow down the flow and creates a way to dissipate the energy gained from going downhill.

Thus whilst the primary energy losing mechanism in the river in the rough uplands is through the surface roughness of the river, the primary mechanism when the average particle size drops to that which provides a relatively smooth bottom – is through “meandering”. Not just from side to side, but also through “sand dunes” on the river bed.

And now there is a balance. If the river becomes too “lossless” in terms of energy loss to vortices – in other words too “smooth”, the water flow increases velocity until it can eat away the bottom or banks – material which is then deposited in bottom dunes, or internal parts of bends to increase the “bendiness” or “roughness” of the river thus enhancing the vortex forming ability of the stream which increases the energy loss per unit travelled.

Ford-Pool sequence

If you read any textbook on rivers, you will quickly learn something about the supposed “riffle-pool” sequence – however since a “riffle” isn’t in my vocabulary and doesn’t seem to have much meaning – and it depends on the state of flow … I’ll call it a shallow-pool or perhaps even ford-pool sequence.
According to this theory all rivers exhibit a sequence of pools interspersed by shallower (fords) at around 6x the length. To be honest I’m not convinced by this theory  on more mountainous streams as it seems to owe much to the fact that a river with a random bottom height will tend to see pools and shallows – and unless you carefully measure the depth – to the casual observer the river is a sequence of (unknown depth) pools and “shallows” – where the water goes over the one point which just happens to be higher than all the other points up to the previous such point so as to create a pool. So, it may not be much shallower than many other places – it just needs to reach that threshold to be the shallowest part of the river within that section.
However, that is not to say that rivers do not show regular longitudinal features. In essences, this is a sequence whereby the water goes through a relatively smooth section – where (particularly in flood) it gains energy – and also tends to pick up particles, followed by a section where the bulk vortices that tend to form in the faster flowing section (particularly at corners or whilst going around obstacles) – begin to create smaller and smaller subsidiary vortices until the whole flow becomes one swirling mass of what quickly turns that into molecular movement we call heat.  This process slows the water and tends to deposit material – thus enhancing the distinction between the deeper pools and shallower “fords” or shallows. Likewise, there is also a change in width of the river. Because the flow along the river must be the same volume, when the river speeds up the area of flow reduces and visa versa, on slower sections the area of flow increases. Thus in order to slow down over the shallows and deposit material, the area of flow must significantly increase. But these are already relatively shallow (because of deposited material), so the width must be significantly greater than average.
This however is what tends to happen at flood – which is the channel forming flows where the river is much higher. But because the added water level is added to the channel profile, during flood there is less distinction between “shallows” and deeps.
Inverting the shallow-pool relationships between flood and droufht
In flood the water is fast through the pools, and slows in the shallows. However, paradoxically, when the water subsides, the pools are then cut off by very shallow shallows. Thus the shallows see a massive change in the flow cross sectional area and the result is that whilst in flood, the shallows tend to be the slower parts of the river, in drier periods, these piles of rocks and other debris form a barrier effectively containing the pools and now the shallows, instead of being slowest in flood, are now the spouts pouring out of the pools ans so the fastest sections of the rivers (aka rapids).
So that the pools (which are faster in flood) become areas of slow moving water in drought, and the shallows (which are slower in flood) with lower water levels become the “lips” of the dams forming the “rapids” in drought.

“Braided” rivers.

As I’ve discussed before (do braided rivers exist) braided rivers are rivers than contain many bottom “sand-dunes” that tend to be on steeper gradient terrain such that the water level often falls below the level at which the braided nature of the river is exposed. In other words, all rivers whose bottoms are composed of particles will tend to form these “sand-dunes” but only those on relatively steep gradients will drain dry enough at low flow to expose them.
Thus the “braided” nature of a river is to some extent a function of the peak to average flow. A river whose flow is always constant, will never expose its “braids”. A dry river, with fine enough sediment to form such features, will always appear “braided” due to the inherent “sand-dune” nature of the bottom.

Width-depth ratio

For obvious reasons as the size of a river increases (i.e. bigger volume of flow), it tends to gain in width and depth. For this reason it is difficult to compare widths, not just between rivers but even from one river to itself after changes like a a tributary joining.
However, whilst width and depth increase, the ratio is not so obviously affected by the size of the river flow.So this metric has been used to try characterise some rivers.
Unfortunately, this is where it all gets hazy, because there doesn’t seem to be a lot of research in this area and that which has been done is sometimes confusing or even contradictory. But it appears from various papers that the width-depth is strongly related to particle size (alpha here is the ratio):

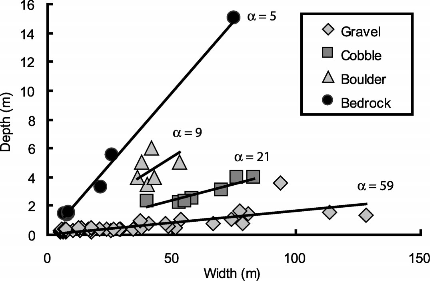
The proviso here is that particle size is itself strongly related to the gradient of a river. So whilst the graph shows an apparent relationship between width-depth ratio and particle size, the physical relationship may be with river gradient (i.e. energy that can be gained per unit moved).
It will also be noticed that the graph does not include finer sediments. I could not find anything giving the whole spectrum, but eventually I found something – but this time it did not include the courser sediments.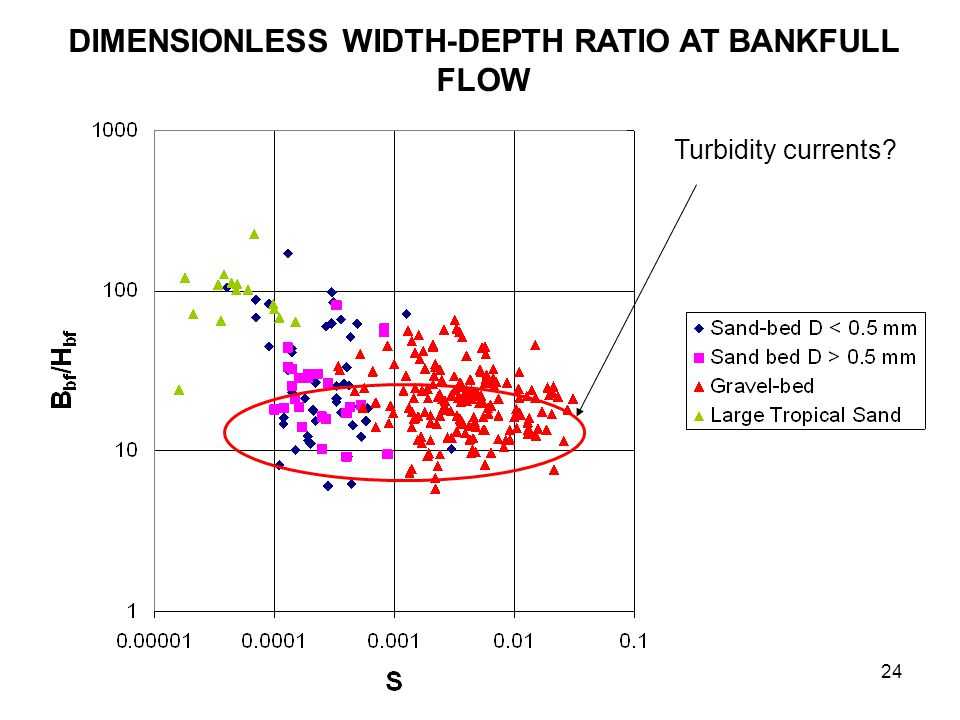
 
However, another proviso: because rivers tend to reduce in gradient & contain finer particles as they get larger, it is difficult to separate out cause and effect.
But between them, this does seem to suggest that the width/depth ratio changes from around 6 (bedrock rivers) to >200 (fine sands and silts). This means that in general, as we go down a river, whilst the flow increases by many orders of magnitude, the width increases much faster than the depth. Thus paradoxically, even relatively large rivers can be relatively shallow – much shallower than we’d assume from the depth of much smaller rivers.
The fundamental reason for this, is that (because the sides of a river are quite small and sloping and can often be ignored) as the river increases in size, the size of the energy absorbing area of the stream needs to increase proportionately if it is to maintain the same speed. But the main energy absorbing contact area is the bottom, so the width needs to increase proportionately to flow to maintain the same flow speed. Thus for the same gradient of stream, in order to flow at the same speed, a much larger river needs to have a proportionately larger width – such that the depth remains constant.
In reality, whilst larger rivers do have a larger width/depth ratio, they are also deeper – which is offset by faster flows (which because turbulence is non-linear with speed have a greater resistance).
To put this another way –  in order to slow down, a river must increase the rough area that a flow is exposed to – so it slows down by widening.  Conversely, a river that deepens, will flow more easily and speed up. These can be self- enhancing. A slowing river deposits more material – tending to broaden out the flow. A fast flowing river can scour the bed, deepening the river.
And in effect, the distance between “slows”, will be the time/distance needed for the river to accelerate to speed, the time thereafter that it takes for large-scale vortices to form, and then for the tide for these large-scale whole-river features to form subsidiary vortices which in turn form further vortices until the whole macro structure (that had formed)is turned into heat.
Of course, occasionally, the features of the river itself create a highly turbulent flow – such as a water-fall. Now, the flow is slowed in the churning as it hits the pool.

Sediment Size

Faster rivers can move larger particles (at least when talking about those larger than sand). As a result, the flow in faster rivers tend to remove much of the fine sediments leaving a bottom “armament” of particles that cannot be easily moved.
Likewise, as a flow slows, the largest particles are the first to be deposited. As a result, the size of the particles along a river can give a good indication of the speed of flow (during channel forming flows).
If however, we return to the pool-shallow sequence, when in flood, the pools are faster flowing so the flows tend to scour their bottoms. Then as the energy is dispelled through vortex formation and slows, the first particles to come out of the flow are the largest, and the size of particles reduces until the slowest part of the flow. Thus we should see a grading of particle sizes along the flow with the smallest at the shallows or “rapids”. Likwise, the particle size tends to increase in the faster “pools” – often becoming bare bedrock in the fastest sections.

Conclusion

The aim of this article was to attempt to describe the various relationships between parameters of a river. It’s beginning to make sense – if you disagree or don’t understand please leave a comment.