Anyone who has done physics, will have heard of viscosity which is usually described in terms like “honey is viscous because it doesn’t flow freely and water is not”.
Fortunately … just the mention of viscosity ought to be enough to put off everyone from reading further – so I make no apologies for the numerous videos which I think were fun!
The problem is that most people have never spotted the glaring anomalies with viscosity. We all accept that atoms in a liquid are in constant movement – yet if they experienced viscosity then as a form of “friction” between particles, it would cause them to all stop moving within a fraction of a second and everything would be a solid. Likewise, we accept that sound waves propagate through a liquid or gas almost unattenuated, but we somehow accept that if instead of being moved toward and back, that if instead the molecules are moved past each other they somehow dramatically change from almost frictionless movement to experience “friction”. That is total absolute bollocks!
I thought if I looked I could find a description of what actually happens at the molecular level – but it’s strangely difficult to find anything on the subject. That often means the “experts” haven’t got a clue which means that as I’ve no reputation to tarnish by admitting how little I know on the subject, I’m quite happy to ask the very awkward question of what viscosity really is.
Introduction
I noticed today that the turbulent velocity of a river flow (the RMS velocity) seems to equal to the energy gained through flowing down the slope of the river. That led me to start wondering how turbulence in open water could cause a resistance to flow – that is to say, that turbulent water must in some way create a back pressure against the flow.
But when I looked to an answer to that question, I found numerous texts explaining flow resistance in terms of viscosity. Not sure if the two were linked I started investigating.
Now, viscosity to me, was one of the black arts of physics. I was never given any clear logical explanation as to why some liquids resisted movement and whilst I accept there are equations that approximate the resistance to movement, it wasn’t something I needed or wanted to know more about so I never sought to look “under the hood” so to speak of the process.
However, now I come to think about it, at a molecular level there is no such thing as “resistance”. Molecules do not touch and to a first approximation they are frictionless points where there must be conservation of energy. So, any energy they gain by being attracted to another molecule, will be EQUALLY LOST when they depart from the vicinity of the other molecule. In other words, if they moved past a series of molecules to a first approximation they might move faster toward other attractant molecules and slower when moving away – but THERE WILL BE ABSOLUTELY NO CHANGE IN ENERGY (if we assume they behave like hard repulsive balls). In simple terms atoms moving past each other would behave like a frictionless roller-coaster – speeding up as they go down the potential well near an attractant molecule, and then slowing down on the other side.
But viscosity is a process that changes movement into heat and in a “fluid” of hard billiard balls with inelastic collisions which is how we model liquids and gases, because the interaction is perfectly elastic, there cannot be any friction.
So, if we consider molecules as “hard”, they must be frictionless, so it must be some kind of departure from this model. So, my first idea was that viscosity occurred rather in the fashion of someone plucking a guitar string. That one molecule would attract another, that that attraction would cause the molecule to “stretch” some kind of bond, rather in the fashion of stretching a guitar string, and then at some point the link would be lost – and the pair would be separated and the molecule that had been stretched would vibrate like a tuning fork. And below is a diagram of a three atom molecule which shows some of the asymmetry which can lead to vibrations which could potentially be “plucked” by a passing molecule.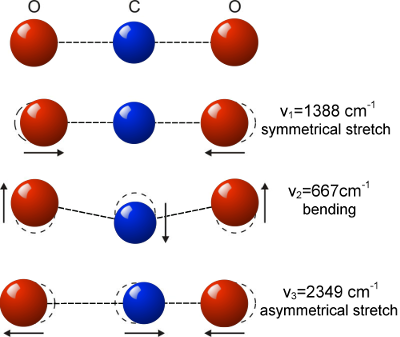
Thus one atom has put energy into the other – but because the “plucking” atom and “pinging” are at a distance after the “pluck” there is no regain of energy as the “plucker” moves out of the potential well. Instead the “plucker” moves away more slowly as its energy was transferred to vibration of the “pinging” molecule.
What led me to this idea is that viscosity is a form of friction and friction is a vector to change movement to heat, and heat is something that molecules like CO2 have a strong interaction with because of their “tuning fork shape”.
That seemed a plausible idea, except it’s total bollocks.
For a start, a single attraction cannot pull a molecule apart – instead there needs to be two opposing forces. For a second, it seems that the stiffest molecules have the highest viscosity which is exactly opposite of what this concept would predict. (link)
So, the evidence shows that the most flexible molecules (the one’s with the stretchiest strings) have the lowest viscosity. The suggestion is that this flexibility, rather than making them “more tangled” as would seem likely actually allows them to “fit in” better and flow more freely than stiff molecules.
That might sound plausible, but then what is the mechanism for translating movement into heat?
I could envisage a more complex system involving at least three molecules, or I could argue that the the second force comes from inertia – but that’s too complex for my simple brain – so like a climate scientist I’m going to claim it’s impossible and move on.
Thus having dismissed vibrational modes as a possible mechanism to convert energy to heat, I know of only one other mechanism, which is that the heat is the movement of the atoms relative to each other. Thus, it must be that viscosity is caused by the movement of one molecule past another causing a translation of their position from the “normal” within the “matrix” that they normally sit, such that the molecule itself is pulled out of position like a guitar string and then “let go” to vibrate within it’s normal “matrix”.
And that would seem a plausible explanation, if you were clueless about gases and liquids which don’t sit in a “matrix” of any form. So, basically that is another bollocks proposal (I think I am on a roll for total bollocks).
Or … perhaps I’m a bit obsessed with guitars?
let’s go back to vortices. Below is a video showing the classic formation of vortices in the wake of a cylinder in a flow:
What this shows, is that vortices – or regions of circular motion form in the wake of objects. At low speeds, two vortices form, at a predictable speed, the pattern changes from double symmetrical vortices to a single vortex which cannot remain stably in the wake, but tends to move to the right allowing a new and opposite vortex to form.
However, what most interests me is that whilst the models predict a love perfectly harmonious swirl, in reality these vortices – which are flowing in opposition to surrounding fluid, give up some of their energy to secondary vortices, and these secondary then shed their energy to tertiary and those to smaller and smaller until the whole movement is a chaotic mass of small scale movement – eventually when we know as “heat”. This video shows the general process quite well:
Thus I don’t think it takes a great leap of faith to see that when vortices start form, they initiate a process of creating smaller and smaller eddies which must inevitably get so small that they will be the movement of single molecules which we know as heat. It is in effect the formation of fractal vortices, that each smaller vortex eventually sheds energy to even smaller vortex, each absorbing energy from the primary initialisation flow as shown in the following video:
And as each subsidiary vortex can only be accelerated by a force, we can simply say that there is a relationship of the form that
v = ∫ F/m dt
Which explains why two flows passing each other lose energy – the energy is being turned into rotation and that rotation then quickly “fractalises” into extremely small perturbations we know as heat.
But what about viscosity?
But whilst this explains how a bulk movement can be turned into the chaotic random movement known as heat, it still doesn’t explain how there can be a back pressure created from (or associated with) the chaos except through viscosity.
And this point I’m completely stuck.
In these situations I often find it informative to “put the cart before the horse”and to find out whether the causation must be the way it seems. In other words, turning around the causation so we explain viscosity as being the result rather than the cause of the turbulent flows.
From That viscosity dragging the liquid moving past and causes rotation.
To that rotation causes the liquid to move past and drag.
And yes … that is as meaningless to me as it probably as to you, which proves that all good ideas have their limits.
Hunches
At this point I know that a simple “push-pull” kind of interaction between molecules is virtually frictionaless and cannot be the cause of viscosity. I also know that it cannot be explained by concepts like “difficult to flow past each other” which only work at a macroscopic level where there is friction between objects – and there is no such thing between molecules. One hunch I have is that a shear is in essence a rotational force and thus one liquid passing another could “ping” the molecules in the other into some form of rotation as demonstrated by this video:
Friction in Solids
However, first lets find out how they explain friction in solids. And what do I find … a video that breaks the conservation of energy!!
The problem with the above video, is that any force experienced by an atom pushing against their “down-motion” neighbour is gained from a reduced force from their “up-motion” neighbour.
Fortunately, the situation would be easily remedied on the video, if the molecules in the lower surface were allowed to move relative to each other and this would then allow the “pinging” effect that I introduced above. This means that there is an asymmetry in the the force such that energy exchanged into molecules down-motion is greater than that gained from those up-motion, similar to this effect:
However, having dealt with friction in solids in a paragraph, I’m back to the problem of viscosity in liquids which aren’t in a matrix where they are “fixed” in such a manner they can vibrate.
let’s have another look at a video of fluids moving past each other for inspiration:
Proposal
At this point I realise that I’ve only got one viable mode of operation whereby I can create an opposing force to one layer of liquid moving past another, which has the necessary “asymmetry” of interactions such that energy is absorbed (rather than movement energy going into compression between molecules and then being perfectly released). In a solid, that asymmetry can occur because atoms/molecules are stuck in a matrix which can be “pinged”. Molecules/atoms in a liquid aren’t in any such matrix. As such the only way energy can be exchanged in a way that allows the “pingee” molecule free to keep the energy is if that energy is converted into rotational motion.
Thus I am proposing that viscosity at the molecular level is an interaction between layers that cause BOTH layers (if liquid) or just the liquid layer to rotate either as individual molecules, or as groups of atoms (a single atom has no asymmetry so an asymmetrical force cannot cause a rotation).
Thus initially, the layers next to the boundary start to move, however, they in turn create a (nearly) equal and opposite movement a the rotational length above them. That then causes another layer to move, but this time in the WRONG DIRECTION {oh &%$£ where’s this going?)
… you can think about it like this (Warning the relevant bit is very short)
So, it seems likely that the physical interaction at the molecular level is that atoms/molecules are caused to rotate, but that you get a strange boundary of contrary rotating layers.
I’m sure at this point it would be sensible to see if this model fits in with the known properties of viscosity – but since it’s my only model I’m stuck with it.
However, I still don’t have a conceptual mechanism to translate all this rotation into heat! Viscosity is fundamentally like friction – a way to convert movement into heat, thus this is pretty fundamental to any conceptual model of viscosity.
However … all I need do, is make the assertion that “flees have flees and those have lesser flees”, and likewise that “vortices shed vortices shed lesser vortices”. As such as the vortices being created get smaller and smaller, at some point the scale of the vortex is that of a single atom and molecule, and now rather than creating a “swirl” it must create a single “impulse” that simply adds to its movement as heat.
Sounds convincing – but again it’s bollocks. Because the “swirl” is a rotational energy and whilst it can’t produce “lesser flees”, neither do we have friction between the molecules to create a vector to change this rotational movement into “vibrational” Brownian type movement which is what we normally think of as heat.
Fortunately, however there’s a principle I can use to hide the fact I’m clueless about how rotational energy gets translated into translational energy and that is that heat fills up all vibrational modes equally. However, that doesn’t explain how rotation changes to translation … only that it must.
So, what I can do, is state that any rotation of anything with asymmetrical charges will create a electromagnetic wave – which is then a vector to take energy from one molecule to another (but not if you believe in the photon theory of light – because it can’t be moved in anything but fixed units – and I would like to find an excuse to explain what happens which doesn’t contradict the “consensus” physics). So, instead, visualise a rotating molecule with an asymmetrical charge. That will cause a successive change of force on neighbouring charged molecules/atoms. That will tend to make them move apart – transferring energy from the rotation to the neighbouring atom. (OK, I’ve now appear to have explained the transfer in a way that doesn’t cite “electro-magnetic waves” – and thus doesn’t appear to conflict with “consensus” physics – except a static potential field is merely the extreme end of the electromagnetic frequency band. And as such it too ought to only transfer energy in discrete packets according to “classical consensus physics”. However, I’m sure if anyone reads this, they’ll remind me that as the frequency approaches zero, the unit energy per photon also approaches zero, so that an infinite number of photons are required to exchange energy in a static field.)
Oh &%$£!!!
So, far I have relied on the opposing mass to generate force. That is to say, force is created by the mass (or more accurately moment of inertia) of the molecules opposing being accelerated.
However, that is all very nice when we start – but viscosity is not a “impulse” but instead a continuous force and that implies that the molecules have to rotate faster and faster and faster.
So, let’s just have a look at that. Let us take a boat moving at 1m/s. Let us then imagine the impact on a water molecule which I read and naively reprint as being “2.75 angstroms“. Which is not much help as I can never remember what an angstrom is. Apparently its 10-10 m or in real money that 0.275 x 10-9 m. Multiply by pi (=3.63 – close enough!) and the circumference is 1 x 10-9 m which means that those molecules in contact with the boat will be spinning at 1 x 109 Hz. Which is in the UHF (TV/WIFI) band and nowhere near as high as I was thinking it might be. If it were anything near normal size an asymmetrical dipole charge would radiate lots of energy, but the very small size of a molecule means that the emissions are small – but no doubt significant because although the atom will not emit much, it’s angular momentum is low so it could be a sizeable chuck over a relatively short time period.
Summary of Viscosity
If I’m right (which I’m probably not), viscosity at the molecular level is a process that drags particles into rotation and this causes the asymmetry of interaction that allows the exchange of energy. However, unlike vortex formation at the macroscopic level, at the molecular level the molecules act as a series of “cogs” – which each successive layer rotating in OPPOSING directions. Likewise, vortex formation is a process that “fractalises” to impart energy from large scale vortices to smaller and smaller ones until the movement is indistinguishable from heat.
Can Turbulence of itself resist flow?
I’d like to propose a rule that the “chaos” of turbulence can exert a force. Instead, what I can say, is that turbulence causes the average speed of movement to be greater than the bulk average flow. Thus any object moving through a turbulent media (including liquids) will experience a more diverse range of forces. If v is the average flow and Δv is the turbulence flow, then because drag is proportional to v2
it is pretty easy to show that the force will increase.
Let’s start with the classic drag equation:
F = ρ CD A v2
Where, ρ is the density of water, CD the drag coefficient (often about 0.5), A the area and v the velocity.
Because when they are in the same direction the force will be proportional to
F = ρ CD A (v+Δv)2 = ρ CD A( v2 +2v Δv + Δv2)
and when the speeds are in opposite directions the force will be
F = ρ CD A (v-Δv)2 = ρ CD A (v2 -2v Δv + Δv2)
So that the average force (for these extremes) is:
F = ρ CD A ((v+Δv)2 + (v-Δv)2)/2 = ρ CD A( v2 + Δv2)
If we then replace Δv with Δ.v and separate out v we have:
F = ρ CD (1 + Δ2) A v2
In other words, if we move through a turbulent flow, the force we experience is as if the fluid had a drag coefficient of C’D = (1 + Δ2) CD
So, whilst turbulence doesn’t create a force, it can effectively change the nature of the flow so that it appears to have a larger drag coefficient. And what is that change doing? It is helping to increase the turbulence which converts translational energy through rotational energy into heat energy.

